| |||||
| How Ptolemy used to calculate both the ascendant and the Part of Fortune. | |||||
|
Since we are going to deal with Hermes' scales and with the directions that Ptolemy explains and exemplifies in the III book of his Quadripartitum, in order to avoid to write a too long and complex article, we will proceed step by step, starting with the calculation of the ascendant and the Part of Fortune. 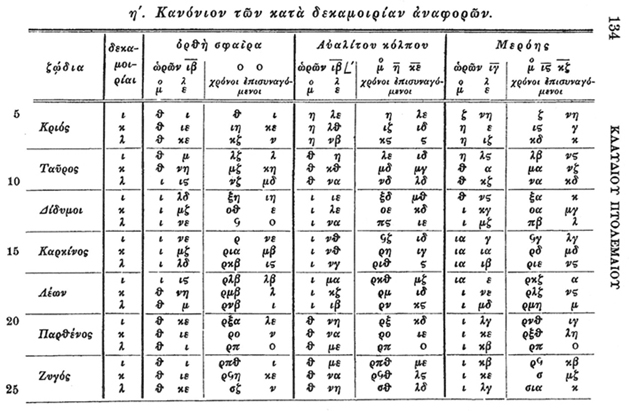 SURPRISING as it may seem, no recent text both of history of astronomy and of astrology, shows the procedure of calculation of the ascendant as it was performed by the Greek-Egyptian astrologers of the first centuries E.V., so that the student of astrology can apply it to his own natal chart. In the second book of his treatise on celestial mechanics Ptolemy proposes a clear table of ascensions. On the first page of this table in the Heiberg edition (here on the right), we see in the various columns (from left): the name of the signs, the degrees of the ecliptic by decans, the respective ascensions on the right sphere (right ascension) and those on the local sphere (oblique ascension) for the first two climates (geographical latitudes); the intermediate values are extracted by proportional interpolation. It must be kept in mind that, when Greek astrologers give examples, they assume that the reader has in front of him a table like that; in other words, when they talk of degrees of the ecliptic, the reference necessarily includes the ascensions of the concerned climate. It is up to the reader to understand if the mentioned degrees are to be referred strictly to the ecliptic or rather to the ascensions corresponding to it. SURPRISING as it may seem, no recent text both of history of astronomy and of astrology, shows the procedure of calculation of the ascendant as it was performed by the Greek-Egyptian astrologers of the first centuries E.V., so that the student of astrology can apply it to his own natal chart. In the second book of his treatise on celestial mechanics Ptolemy proposes a clear table of ascensions. On the first page of this table in the Heiberg edition (here on the right), we see in the various columns (from left): the name of the signs, the degrees of the ecliptic by decans, the respective ascensions on the right sphere (right ascension) and those on the local sphere (oblique ascension) for the first two climates (geographical latitudes); the intermediate values are extracted by proportional interpolation. It must be kept in mind that, when Greek astrologers give examples, they assume that the reader has in front of him a table like that; in other words, when they talk of degrees of the ecliptic, the reference necessarily includes the ascensions of the concerned climate. It is up to the reader to understand if the mentioned degrees are to be referred strictly to the ecliptic or rather to the ascensions corresponding to it.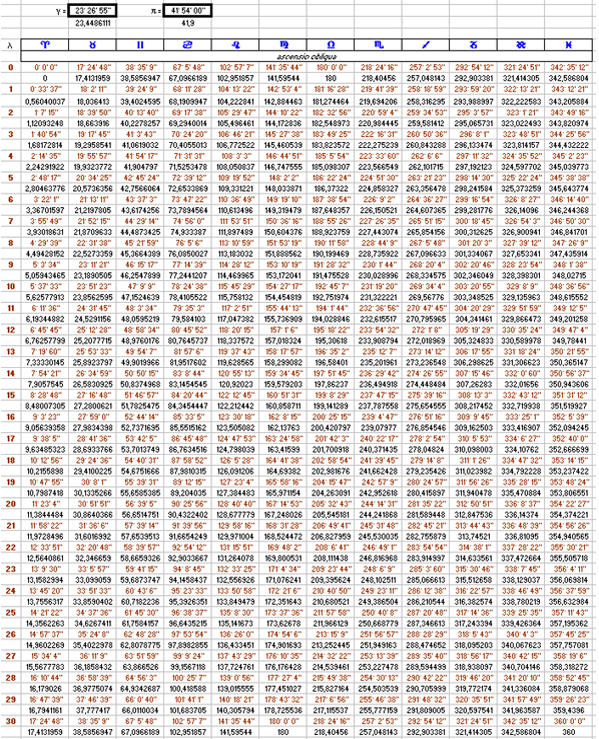 Since today we have a program accessible to all those who have a computer, i.e. Excel, with which we can perform all the necessary calculations, the student of astrology should prepare a similar table (we give an example here on the left). After having chosen two cells (in the prospectus the two cells at the top), one for ε (the size of the angle γ) and one for the pole φ (the geographic latitude, in the prospectus indicated by π), insert vertically the degrees of the ecliptic in the first column on the left, one for each row, and horizontally at the top the twelve signs: then it will be sufficient to put in each cell the formula that allows to calculate the oblique ascension of each degree of the ecliptic, which will constantly draw ε and φ from their cells. In this way you have only to change in the two cells chosen the values of ε and φ, to see all the new ascensions calculated. Such a table can then be extended to include not only the right ascension (symbol α), but also the size in equinoctial degrees of the unequal hour of day and night, and the maximum length of the day. Although Excel can use the 'Function Convert_Degree' and the 'Function Convert_Decimal' to switch between sexagesimal and decimal degrees, the calculations are based on decimal degrees. The ideal solution is to dedicate a pair of cells to each piece of data: below, the one with the decimal degree (in black in the prospectus), for calculations; above, the one with the sexagesimal degree (in brown). It is preferable not to cut off the decimals, leaving at least seven. After all, the astrologers of the early centuries made use, in addition to seconds, also of thirds, quarters and more (see our translation of Book I of the Almagest, p. 29 n. 30). Since today we have a program accessible to all those who have a computer, i.e. Excel, with which we can perform all the necessary calculations, the student of astrology should prepare a similar table (we give an example here on the left). After having chosen two cells (in the prospectus the two cells at the top), one for ε (the size of the angle γ) and one for the pole φ (the geographic latitude, in the prospectus indicated by π), insert vertically the degrees of the ecliptic in the first column on the left, one for each row, and horizontally at the top the twelve signs: then it will be sufficient to put in each cell the formula that allows to calculate the oblique ascension of each degree of the ecliptic, which will constantly draw ε and φ from their cells. In this way you have only to change in the two cells chosen the values of ε and φ, to see all the new ascensions calculated. Such a table can then be extended to include not only the right ascension (symbol α), but also the size in equinoctial degrees of the unequal hour of day and night, and the maximum length of the day. Although Excel can use the 'Function Convert_Degree' and the 'Function Convert_Decimal' to switch between sexagesimal and decimal degrees, the calculations are based on decimal degrees. The ideal solution is to dedicate a pair of cells to each piece of data: below, the one with the decimal degree (in black in the prospectus), for calculations; above, the one with the sexagesimal degree (in brown). It is preferable not to cut off the decimals, leaving at least seven. After all, the astrologers of the early centuries made use, in addition to seconds, also of thirds, quarters and more (see our translation of Book I of the Almagest, p. 29 n. 30).As an example of a celestial chart, we will use Ennio Morricone's one, already the subject of our short article entitled About the natal chart etc., where you find the data taken from SolFire (SF).
1. Well, to calculate the degree rising at a given time of day or night in a given place, it is necessary first to know the size of the unequal hour of day or night, which is obtained from the position of the Sun on the ecliptic in the following way: from the tables we calculate by proportional interpolation, if necessary, the α and the oblique ascension (symbol αφ) of the place on the ecliptic occupied by the Sun at the given hour: their difference is called ascensional difference (Δa), whose sixth added to or subtracted from 15° will give the size of the unequal hour.[1] In the case of E. Morricone the Sun has a longitude (λ) of 228° 15' (i.e., 18° ♏ 15'), to which corresponds an α of 225° 47' 16” and an αφ of 241° 59' 06”, the difference of which is 16° 11' 50”; the sixth of 16° 11' 50”, i.e. 2° 41' 58”, added to 15° will give 17° 41' 58”, which is the size of the unequal nocturnal hour expressed in equinoctial degrees.
It is possible to proceed in another way. After having measured the αφ of the place of the Sun, let us also measure the αφ of the opposite place, that is 18° ♉ 15', which will result to be 29° 35' 25”. The difference between the two αφ, being the Sun below the horizon, will give the length of the night in equinoctial degrees, the twelfth of which will be the size of the unequal hour: namely, 241° 59' 06” – 29° 35' 25” = 212° 23' 41”, which divided by 12 gives 17° 41' 58”, talling with 1h 10m 48s.
2. Once the size of the single unequal night hour has been found, it must be multiplied by the time of the event, which, however, we moderns no longer record. It is therefore necessary to turn the local time of birth into the corresponding unequal hour of the night. Let us therefore convert Greenwich Mean Time into local time: 22h 25m –1 (time zone) + 49m 56s (long. E), i.e. 22h 14m 56s. But since the local time must be the true one, not the mean one, we have to remove the equation of time: 22h 14m 56s – 15m 59s = 21h 58m 57s.
3.
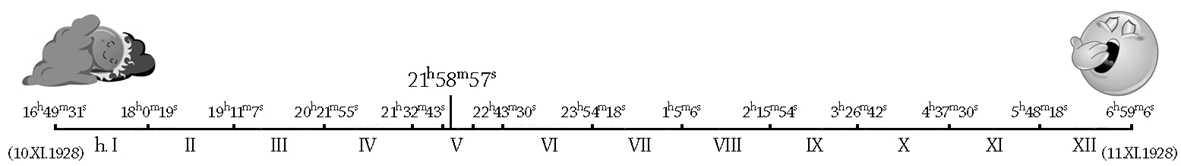 According to SF, on November 10, 1928 the Sun sets at 16h 49m 31s and the following day it rises at 6h 59m 18s. Now we add up to the hour of sunset the unequal night hour (1h 10m 48s) 12 times until we reach the time of sunrise of the subsequent day, which will be at 6h 59m 6s, i.e. slightly lower (by 12s) than the time indicated by SF (this difference is due to averages, truncations, rounding up and down, and other causes of various origin). The result is the following series (see diagram): the first hour of the night goes from 16h 49m 31s to 18h 00m 19s; the second from 18h 00m 19s to 19h 11m 07s; the third from 19h 11m 07s to 20h 21m 55s; the fourth from 20h 21m 55s to 21h 32m 43s; the fifth from 21h 32m 43s to 22h 43m 30s. And here we stop, because we have already passed the true local time of birth. We can therefore establish that the birth took place after the beginning of the fifth hour (21h 32m 43s). According to SF, on November 10, 1928 the Sun sets at 16h 49m 31s and the following day it rises at 6h 59m 18s. Now we add up to the hour of sunset the unequal night hour (1h 10m 48s) 12 times until we reach the time of sunrise of the subsequent day, which will be at 6h 59m 6s, i.e. slightly lower (by 12s) than the time indicated by SF (this difference is due to averages, truncations, rounding up and down, and other causes of various origin). The result is the following series (see diagram): the first hour of the night goes from 16h 49m 31s to 18h 00m 19s; the second from 18h 00m 19s to 19h 11m 07s; the third from 19h 11m 07s to 20h 21m 55s; the fourth from 20h 21m 55s to 21h 32m 43s; the fifth from 21h 32m 43s to 22h 43m 30s. And here we stop, because we have already passed the true local time of birth. We can therefore establish that the birth took place after the beginning of the fifth hour (21h 32m 43s).4. The difference between the true local time (21h 58m 57s) and the beginning of the fifth hour (21h 32m 43s), which is 26m 14s, must be adjusted, before multiplication, to the unequal hour, so that 30m 58s of unequal hour will turn out to correspond to 26m 14s. Such an adjusted difference shall be expressed in fractions, i.e. 1/3 + 1/10. Thus, to the hour of birth expressed in Greenwich Mean Time, that is 22h 25m, corresponds the 4th hour of the night + 1/3 + 1/10 with a slight rounding down.
5. Now we can multiply the unequal nocturnal hour (17° 41' 58") by the hours elapsed from the beginning of the night (4h +1/3 [=23m 36s] + 1/10[=7m 5s]) and we obtain 79° 55' 55".
6. All that remains is to add the results of points 1. and 5. to get the αφ of the ascendant: 29° 35' 25” + 79° 55' 55” = 109° 31' 21”.
Recalling that the right ascension of the true MC (see the cited article) is 19° 31' 16", and that therefore the αφ of the horoscope is 109° 31' 16", we can compare the two results.[2]
Theon Alexandrinus in his concise commentary on Ptolemy's tables summarises the procedure as follows: "When the hour is diurnal, we multiply the hourly times corresponding to the degree of the Sun in the ascension table under the appropriate climate and sign by the unequal hours passed from sunrise. To the result we add the ascension times corresponding to the same degree; we carry the sum of the times—subtracting 360° if necessary—in the same climate as the ascensions. Where the number falls in the column of ascensions, we will say that the sign containing the degree and the degree itself that in the grid is on the same line, rise or are the horoscope".[3] Very clear!
We can rightly conclude that—unlike those who, in order to conceal their ignorance, project it onto the ancients—the astrologers of the first centuries knew how to make calculations and were very precise.
And let us move on to...
...the Part of Fortune. THE NONSENSE that has been peddled for centuries about how to calculate the Part of Fortune has no justification. Already in ancient times the error had crept in; however, since the difficulty of finding competent astrologers or of finding and consulting or buying reliable texts was almost insurmountable in the early centuries, a justification can be given. For modern scholars, no, because the only justification can be given by a mixture of incompetence, ignorance and arrogance: rather than admitting they do not understand—which is not a sin, but a virtue—, academics, and not only, prefer to ignore what they do not understand, and project their own inadequacy on the ancients, or—which is worse—they take for granted and certain what is not, i.e. they lie!
Before Ptolemy the calculation of the Part of Fortune is explained by Manilius (3,186÷196). The poet says: when you will have ascertained certo discrimine, "with a sure division (of the sky)", if the birth is diurnal, a sole ad lunam numerabis in ordine partes signorum, "you will count the parts of the signs [= the degrees], according to their order, from the sun to the moon", ortivo totidem cardine duces, "(and) you will carry them from the eastern pivot", quem bene partitis memorant[4] horoscopon astris, "which, once well divided the sky, they call horoscope"; in quodcumque igitur numerus pervenerit astrum, hoc da Fortunae, "in whatever point of the sky, therefore, the (computed) number arrives, assign it to Fortune"; but when the birth is nocturnal, verte vias, sicut naturae vertitur ordo, "reverse the way, as when the natural order is reversed". About four centuries later, Paulus Alexandrinus proposes the same procedure again.[5] This is a simplified calculation, as it were, the source of which could be found among those astrologers who misunderstood Nechepso and Petosiris (see below) or did not accept their principle.
The most important text in terms of authority is that of Ptolemy. The acute intelligence of the man and the incomparable competence of the scholar outweigh all the writings of his colleagues and impose extreme caution on his epigones. First of all, it should be noted that Ptolemy does not speak about the Part of Fortune in a context dedicated to the lots, and it is therefore incorrect to affirm—as Feraboli does (l.c., p. 262)—that "of all κλῆροι Ptolemy will keep only the Part of Fortune for a pure need of geometric correspondences" (but what does it mean?!). Ptolemy treats the Part of Fortune in the context of life givers, because it can assume in certain circumstances the role of apheta. Ptolemy says (3,10,5): "... the Part of Fortune is that place which is drawn by counting, in any case (πάντοτε) both by day and by night, from the sun to the moon, carrying the same amount from the horoscope in the direction of the following signs, so that that ratio and (that) configuration the sun has with the horoscope, the moon also has to the Part of Fortune, which is as a lunar horoscope. {Most likely the writer this wants to mean: (you have) to compute, for those born at night, from the moon to the sun and to throw back from the horoscope, that is in the direction of the preceding signs; and so, in fact, the same Part of Fortune and the same configuration will result}".[6] Ptolemy will reiterate the concept in the book IV (2,1): "As for the possessions, whatever will be their entity, they are detected by the so called Part of Fortune, the only one (μόνου
μέντοι) whose distance in any case from the sun to the moon we raise from the horoscope both for those born by day and by night, for the reasons we said dealing with the years of life".
The third text is drawn from Vettius Valens, a professional astrologer contemporaneous of Ptolemy. In book III we find a small chapter (14 K., 11 P.) dedicated to the Part of Fortune, in which the author confesses: "I found that the ancients have elaborated the procedure related to the years of life in a (too) complicated way; I believe, however, after careful research, that I do not displease most of (my) readers. In the 13th book the King [= the Venerable] after the proem and the orderly exposition of the signs passes to the Part of Fortune (which is extracted) from the Sun, the Moon and the horoscope; lot that he elevates to great importance and mentions throughout the book, and judges authoritative its place, about which he does not let us distinguish well (what he means by) 'in reverse' and 'backwards' (τὸ ἔμπαλιν
καὶ ἀνάπαλιν): 'And the Sun (—he writes—) starting from dawn, while ending the vespertine arc, leaves (παραδίδωσι) the vault of the eternity of time, as we can see; nevertheless at the coming of the night the Moon will not always be bearer of light, but sometimes, appearing in the evening, it sets; sometimes it remains for a certain stretch, and other times it will be seen crossing the whole night. It follows therefore that it entrusts totally to the Sun its round'. In this regard, some people think in one way, others in another; I am of the idea of taking, in a diurnal birth, from the Sun to the Moon and the same from the horoscope, but in a nocturnal one, as long as the Moon is above the Earth, that is until it sets, to take from it to the Sun and the same from the horoscope and, after sunset, from the Sun to it.[7] The addition, in fact, 'therefore it entrusts totally to the Sun its round', seems (to mean) just this".[8]
Valens, however, does not quote here the passage in which ἔμπαλιν and ἀνάπαλιν are to be found. Had he forgotten that? We do not think so: he shows the text which would support his interpretation. Actually, in the third chapter of Book II he writes: "Wanting to verify more accurately the argument concerning happiness, I turn to the lot of fortune which is the most necessary and powerful place, as also the King in the 13th book began to expound in an occult way by saying: 'And afterwards it will be necessary for the day-born to count accurately from the Sun to the Moon, but in reverse (ἔμπαλιν δὲ) (it will be necessary) to assign equality from the horoscope (ἀφ'ὡροσκόπου ἰσότητα
τάσσειν) [...]'. Similarly Petosiris explained the place in his Terms. Others treat it in a different way, and we shall also expound it in due course, with other commented ways related to the topic of happiness." Indeed, the King's text seems obscure, but not completely incomprehensible: its understanding is based entirely on the meaning of ἔμπαλιν δέ, which the translators have quite misunderstood (they translate 'back' and 'in the opposite direction') and make a synonym of ἀνάπαλιν of it! A good example of the semantic value of ἔμπαλιν is offered by Dorotheus talking about marriage (P. 393,23): '(If) Venus is in a masculine sign and Jupiter in a feminine one, such a configuration is favourable for males; the reverse (ἔμπαλιν δὲ) for females, (i.e.) when Venus is in a feminine sign and Jupiter in a masculine one'. In the passage quoted by Valens, therefore, ἔμπαλιν means 'counting in reverse, i.e. from the Moon to the Sun'; and it is precisely ἔμπαλιν which allows to understand the following, i.e. “to assign equality from the horoscope”: whether you count from the Sun to the Moon, or from the Moon to the Sun, from the horoscope (in one direction or the other) there must be equality of result. Which agrees with the phrase 'it totally entrusts its round to the Sun': in other words, while the Sun leaves the celestial vault at sunset, the Moon does not and cannot do the same. The movement of both stars on the zodiac is only counterclockwise, i.e. in the direction of the following signs; and, since the Moon constantly returns to join the Sun, its phases depend exclusively on the diurnal luminary. Hence, their ratio is determined by the Sun—without which the Moon would be a dull body—and always in the direction of the following signs. Frankly, we do not believe that Valens, a professional and capable astrologer to be sure, did not understand the meaning of King's words, rather he was not convinced—in fact he also calls into question ἀνάπαλιν which is not in the quoted passage (did he want to force the meaning of ἔμπαλιν?)—; and he did not want to take the astronomical parallelism between Sun-horoscope and Moon-Part of Fortune into account.
The fourth text is that of the Anonymous Commentator. Unfortunately, there is no modern edition of this commentary and the only one available dates from 1559. In the preface, its editor[9] tells his readers that, disappointed by the Latin translation of this commentary, falsely attributed to Valla, and having found the codex which it was based on, he asked a very competent friend to check the version and amend the errors. His friend, however, having collated the first page, found such a mass of errors that the margins were not sufficient to contain all the corrections. So the editor asked him to translate the commentary himself. His friend, although busy, completed the work, not without remarking that he had never had such a heavy job: in fact, the Greek writing was very bad, very difficult to read; moreover the text of Ptolemy and that of the Commentator were confused, and the work itself was so corrupt and mutilated that it was impossible to extract a complete sentence. In the end, his friend thanks to his own intuition and expertise managed to recompose a text that was in some way useful to scholars, and the work was delivered on one condition: that the author's name not be mentioned. Actually, even the printed text, as reassembled, is not easy to read: the spelling errors are many; the syntax indefinable; the confusion between numbers and symbols is disorienting; and the Latin translation sometimes tries to reinterpret the Greek. Perhaps it is for these reasons that almost no one reads this commentary, which, in spite of everything, is undoubtedly the most important aid, written by a very capable and extremely competent astrologer. The text is arranged as follows: the Commentator quotes a few words from the Ptolemaic text as a paragraph heading, followed by his observations.
Well, at 3,11,5 (μετὰ δὲ
ταῦτα...) the Anonymous (Wolf 111) comments, among other things: ".... It is necessary... to know that the Venerable[10] does not assume the Part of Fortune as others do, but always calculates from the Sun to the Moon, and (put) the same parts from the horoscope. In fact (Ptolemy) says that (the others) have not understood the writings of the Venerable about this lot[11], and by Venerable he alludes to Nechepso and Petosiris: they are in fact the first ones to have deployed the prediction by means of astrology. What did they say then? (Well,) when you take the lot of Fortune, by day count from the Sun to the Moon and raise similar parts from the horoscope according to the direction of the following signs of the zodiac; by night, the reverse. But what does the reverse mean?[12] In order to do (the reverse, i.e.) from the Moon to the Sun, you will never raise in the direction of the following signs, but in the direction of the preceding signs. (In this way,) in fact, you find again the same (place) that was computed before from the Sun to the Moon. Those who came afterwards did not keep the whole (procedure) of the reverse, but (limited themselves) to the computation from the Moon to the Sun, (i.e.) they did not do the reverse (operating also) in the direction of the preceding signs. That the Part of Fortune is the nocturnal and lunar horoscope, is clear from what the Venerable writes:[13] in fact the same ratio in degrees and configuration that the Moon will have with the lot of Fortune, has the Sun with respect to the horoscope". Two points must be underlined here: 1. whether it is calculated from the Sun to the Moon or from the Moon to the Sun, the place must be the same; 2. the Part of Fortune must have with the horoscope—not the contrary—the same ratio and configuration that the Sun has with the Moon. The addition of the configuration (σχηματισμός) to the ratio (λόγος) shuts down the question, because the inversion of the places would modify the configuration. And the ratio (λόγος) can only be based on hours. If we were to operate on the ecliptic, the local sphere would droop and flatten on a horizontal plane!
The Anonymous then continues: "It is obvious, just from these things (that have been said), that it will be better if we use the same method, the one by means of the tables, that (we used) also for the horoscope: the Part of Fortune, in fact, will be found in the way (we found) the hours [= the horoscope]. By carrying (in the tables), in fact, for diurnal births the degree of the Moon—for nocturnal ones the diametrically opposite degree—and detected the ascending times, (we) multiply them by the hours; and the resulting number we add to the anaphora; then we will look up in the same climate, where that number falls and we say that there the lunar horoscope is placed".
1. Placing the Moon on the ecliptic at 1°♏46'12” (this datum does not correspond perfectly to that given by SF, because we interpolate it by our module), we can see from the ascension table that the corresponding αφ is 219° 19' 32”, and the unequal diurnal hour holds 13° 31' 43”. Now, if we compute from the Sun to the Moon, we would have to make almost the entire tour of the zodiac; therefore, it is much easier to compute from the Moon to the Sun and then to bring back the data from the horoscope in the direction of the previous signs, i.e. in reverse (ἔμπαλιν).
2. We have seen above that the Sun is at the fourth hour + 1/3 + 1/10 of the night. Let us multiply, then, the unequal daytime hour of the Moon × 4,5160494 and we will obtain 61° 05' 47”.
3. Let us add the result to the αφ of the Moon: 61° 05' 47” + 219° 19' 32” = 280° 25' 19”. Since we have chosen to simplify the calculations, we must pass to the opposite place: 280° 25' 19” – 180° = 100° 25' 19”. But this is not yet the αφ of the Part of Fortune, because, being deviated from the horoscope, its pole is in any case lower than the geographical latitude. However, the Anonymous Commentator, who was well aware of the problem, recommends to search for the found number "in the same climate (ἐν τῷ αὐτῷ
κλίματι)», then neglecting to indicate how to proceed with the adjustment: he almost certainly took it for granted. Besides, since astrologers and astronomers worked with anaphoras, unequal hours and day length, the adjustment could be considered an unnecessary detail.
4. Having already found the αφ of the horoscope (109° 31' 16”), let us make the subtraction: 109° 31' 16” - 100° 25' 19” = 9° 5' 57”; and let us find the difference between the equinoctial hour and the diurnal hour of the Moon: 15° – 13° 31' 43” = 1° 28' 17”. By a simple proportion we can get the size of the portion of 15° corresponding to 9° 5' 57”, which will be 10° 5' 20”, whose difference (10° 5' 20” – 9° 5' 57” = 0° 59' 23”) will give us the size of the adjustment. To confirm this, we can proceed as follows: since 9° 5' 57” matches 40m 21s of the unequal diurnal hour of the Moon, noting also from our speculum that the Δa of the Moon is 8° 49' 39”, we will find the size of the adjustment from the following proportion: 8° 49' 39” : 6h = x : 40m 21s, where x will be equal to 0° 59' 22”.
5. Since we have chosen the opposite procedure, we shall add the adjustment to the αφ previously obtained: 100° 25' 19” + 0° 59' 22” = 101° 24' 41”, which is the αφ of the Part of Fortune corresponding to 28° ♋ 47'06”.
Today, with all the aids we have at our disposal, we can simplify the whole procedure simply by operating with the αφ/κφ (oblique descension) of the luminaries. In Ennio Morricone's chart, we will deduct the difference between the oblique descension of Sun and Moon (209° 35' 26” – 201° 40' 13” = 7° 55' 13”) from the αφ of the horoscope: 109° 31' 16” – 7° 55' 13” = 101° 36' 03” corresponding to 28° ♋ 56'04". The discrepancy between the result obtained with the ancient method and the modern one depends on various causes.[14]
It remains only to verify if their distances agree: κφ Sun – αφ horoscope (209° 35' 26” – 109° 31' 16”) = 100° 04' 10”; κφ Moon – αφ Part of Fortune (201° 40' 13” – 101° 36' 03”) = 100° 04' 10”. And these are the degrees that must agree, not those on the ecliptic!
This is the only method to find the true place of the Part of Fortune, and, as far as we know, the only astrologer to apply it correctly was John Worsdale, who explains the procedure in his Celestial Philosophy, London 1828, pp. 17÷18.
____________________________
NOTE [1]
Following the example of some scholars of the history of astronomy, the unequal hour is commonly referred to as the seasonal hour, but this is not correct, since there are no hours valid for an entire season, since each unequal hour is only equal to itself due to each hour being different from every preceding or following one. It is only for the sake of convenience of calculation that we divide the space of time from sunrise to sunset, and vice versa, into 12 equal hours. In fact, the Greek adjective καιρική, as an attribute of ὥρα, does not mean 'seasonal', but 'of that moment' 'temporary', i.e. specific to that moment and only to that moment. However, since we equate both the 12 hours of day and night, we will call them 'unequal'.
[2]
The difference is trifling here, but see note 14.
[3]
Cf. the splendid edition Le “Petit Commentaire” de Théon d'Alexandrie aux Tables faciles de Ptolémée, ed. Anne Tihon, Città del Vaticano (Bibl. Apost. Vaticana) 1978, p. 219.
[4]
For the meaning here of memorare, cf. Pacuv. 89: id quod nostri caelum memorant, Grai perhibent aethera, "what ours call caelum, the Greeks (call) aethera".
[5]
Cf. Pauli Alexandrini Elementa apotelesmatica, ed. Æ. Boer, Lipsiae 1958, p. 47 s.; Manilio, Astronomica, comm. a cura di S. Feraboli e R. Scarcia, II, Milano 2001, p. 263): Feraboli does not seem to have clear ideas about this point.
[6]
The author of this gloss, which Hübner surprisingly accepts in the text, makes the same mistake as Manilius does by separating diurnal from nocturnal births, even though he then upholds the identity of the place computed in the direction of the following signs with the one computed in the direction of the preceding signs: either the gloss is irretrievably corrupt, or the author does not realise the obvious contradiction. Moreover: 1. ἴσως, 'likely', contrasts according to logic with πάντοτε of the previous paragraph; 2. the construction of the sentence's beginning is rather convoluted; 3. at the beginning of the chapter Ptolemy alludes to Nechepso with ὁ
ἀρχαῖος not συγγραφεύς; 'writer'; 4. Ptolemy never uses the infinitive of διεκβάλλω (not that of ἐκβάλλω either); 5. finally, in Proclus' Paraphrase this paragraph is ignored. We have enclosed the text in braces to indicate that it has to be expunged, as it is undoubtedly a gloss extraneous to Ptolemy.
[7]
Feraboli, l.c., makes Valens say exactly the opposite!
[8]
Some translators seem to rely more on their imagination than on the Greek text: they translate ἔμπαλιν as 'forward' and παραδίδωσι as 'opens'!
[9]
According to CCAG (VIII,2 p. 5) the editor is Hieronymus Wolf—but in the printed edition consulted there is no trace of the name, and Hübner does not mention him either (cf. p. LII of his Ptolemaic edition, where he writes ἐξήγησις instead of ἐξηγητής)—and the text was based on cod. Paris. Gr. 2411. However, in the dedicatory preface to the treatise Hermetis philosophi De revolutionibus nativitatum, incerto interprete (pp. 205÷279) the editor, addressing Onoratissimo Viro, Paulo Heintzelio Patricio Augustano, signs himself as Hieronymus Vuolfius (in the first lines of p. 208, he then wittily notes in passing that sunt qui me λύκον graece, quam Vuolfium barbare nominare malint, “some people prefer to call me Lykon [= Wolf] in a Greek way rather than Wolf [=Wolf] in the barbarian tongue”).
[10]
We translate ὁ παλαιός with 'the Venerable', although it means 'the Old', since Ptolemy, at the beginning of the chapter refers to him with ὁ ἀρχαῖος, literally 'the Ancient', which we translate with 'the Venerable', since the use of ἀρχαῖος referring to persons may allude to an authoritative personality, the former, no longer equalled. The Latin translation confuses him with Ptolemy. Riess, who edited the fragments of Nechepso and Petosiris (cf. “Philologus” VI Supplementband 1891-1893, pp. 327÷394), unbelievably substitutes, in quoting the excerpt, ὁ παλαιός with ὁ
Πτολεμαῖος, without realizing that shortly after the Anonymous specifies: λέγει δὲ παλαιὸν τὸν Νεκεψὼ
καὶ Πετόσιρον [sic!]!
[11]
Here the Anonymous extends what Ptolemy says, who merely observes that "according to the Venerable, it is laughable to lay every future fact on an individual, who, because of the years of life allotted to him, will never reach the age at which those fact should occur". Such an extension, however, is not nonsensical.
[12]
We point out that the Anonymous Commentator does not use ἔμπαλιν, but ἀνάπαλιν. It is not a real error, but it may cause a misunderstanding: ἔμπαλιν emphasizes the switching, ἀνάπαλιν generically the opposite.
[13]
Although it is almost obvious from the quotation of the Venerable that Ptolemy, while dealing with the years of life, follows him, there is no direct reference in his own exposition. Here the Anonymous Commentator, quoting the Venerable, seems to want to uphold Ptolemy. In any case, unlike Valens, the Anonymous has no doubts about the interpretation of the text of the 'King'-Venerable.
[14]
The two calculation procedures, ancient and modern, can hardly agree due to the imprecision of the data not only on the equation of time, sunrise and sunset times, truncations and/or decimal approximations, but also on planetary positions, especially of the Moon. For example, the Meeus-Ferrari module, available online, for the calculation of sunrise and sunset gives slightly different times. However, using the values given by SF as the basis for our comparisons and check, we must stick to those.
[Dorno, June 6, 2022]
| © Franco Luigi Viero  | ||||
